Page 4 - CH-7-SLRC-HOME ASSIGNMENT

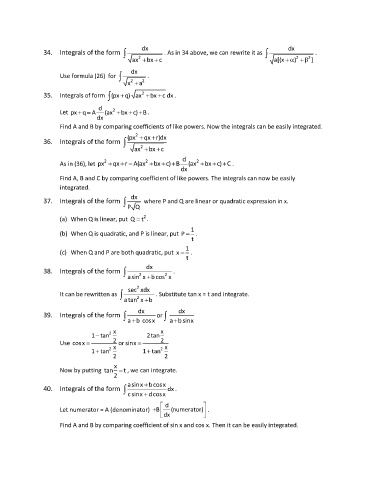
34. Integrals of the form  dx . As in 34 above, we can rewrite it as  dx .
2
ax  2 bx c a[(x    2 ]

)
Use formula (26) for  dx .
x  a 2
2


2
35. Integrals of form (px q) ax  bx c dx .

 
 
2
Let px q A d (ax  bx c) B.
dx
Find A and B by comparing coefficients of like powers. Now the integrals can be easily integrated.
(px  2 qx r)dx

36. Integrals of the form 
ax  2 bx c

 
2
As in (36), let px  qx r A(ax  bx c) B d (ax  bx c) C .
 
2
 
2
dx
Find A, B and C by comparing coefficient of like powers. The integrals can now be easily
integrated.
37. Integrals of the form  dx where P and Q are linear or quadratic expression in x.
P Q

2
(a) When Q is linear, put Q t .
1
(b) When Q is quadratic, and P is linear, put P  .
t
1
(c) When Q and P are both quadratic, put x  .
t
38. Integrals of the form  dx .

2
2
asin x bcos x
2
It can be rewritten as  sec xdx . Substitute tan x = t and integrate.

2
atan x b
dx
dx
39. Integrals of the form  a b cosx or  a bsinx



1 tan 2 x 2tan x
Use cosx  2 or sinx  2


1 tan 2 x 1 tan 2 x
2 2
x
Now by putting tan  , we can integrate.
t
2

40. Integrals of the form  asinx bcosx dx .

csinx dcosx
 d 
Let numerator = A (denominator) B   dx (numerator) .


Find A and B by comparing coefficient of sin x and cos x. Then it can be easily integrated.