Page 3 - CH-7-SLRC-HOME ASSIGNMENT


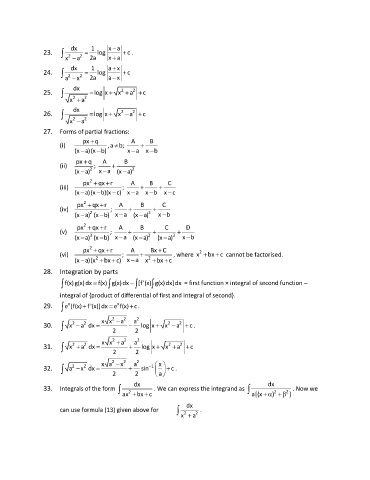
23. 2  dx  1 log x a  c .

x  a 2 2a x a

24. 2  dx  1 log a x  c
a  x 2 2a a x

25.  dx  log x  x  a 
2
2
c
2
x  a 2
2
26.  dx  log x  x  a 
2
c
x  a 2
2
27. Forms of partial fractions:

px q A B

(i) ,a b; 




(x a)(x b) x a x b

px q A B
(ii) ; 



(x a) 2 x a (x a) 2

px  2 qx r A B C
(iii) ;  






(x a)(x b)(x c) x a x b x c
px  2 qx r  A B C
(iv) ;  




2
(x a) (x b) x a (x a) 2 x b
px  2 qx r  A B C D
(v) ;   






3
(x a) (x b) x a (x a) 2 (x a) 3 x b
px  qx r  A Bx C

2

(vi) ;  , where x  2 bx c cannot be factorised.

(x a)(x  bx c) x a x  bx c

2


2
28. Integration by parts
 f(x)g(x)dx f(x) g(x)dx    [f'(x) g(x)dx]dx = first function × integral of second function –


integral of {product of differential of first and integral of second}.


29.  e [f(x) f'(x)]dx e f(x) c.
x

x
x x  a 2 a 2
2
2
30.  x  a dx   log x  x  a  .
2
2
2
c
2 2
2
2
2
2
31.  x  a dx  x x  a 2  a 2 2 log x  x  a 
2
c
2
2
2
2
32.  a  x dx  x a  x 2  a 2 sin  1   x    c .
2 2  a 
33. Integrals of the form  dx . We can express the integrand as  dx . Now we
2
ax  2 bx c a[(x   2 ]

)
can use formula (13) given above for 2  dx .
x  a 2