Page 1 - CH-7-SLRC-HOME ASSIGNMENT

INTEGRALS

LESSON NOTES

Integration is the inverse process of differentiation. Instead of differentiating a function, we are
given the derivative of a function and asked to find its primitive, i.e., the original function. Such
a process is called integration or anti differentiation.

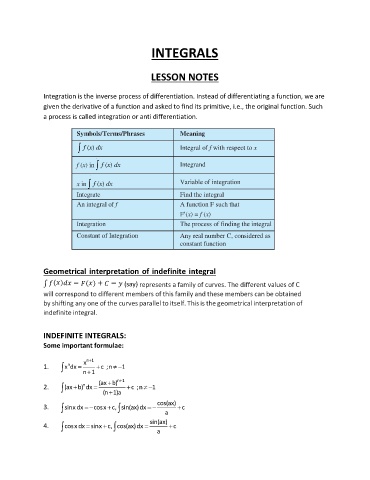
Symbols/Terms/Phrases Meaning
∫ f (x) dx Integral of f with respect to x

∫
f (x) in f (x) dx Integrand

∫
x in f (x) dx Variable of integration
Integrate Find the integral
An integral of f A function F such that
F(x) = f (x)
Integration The process of finding the integral

Constant of Integration Any real number C, considered as
constant function

Geometrical interpretation of indefinite integral

∫ ( ) = ( ) + = (say) represents a family of curves. The different values of C
will correspond to different members of this family and these members can be obtained
by shifting any one of the curves parallel to itself. This is the geometrical interpretation of
indefinite integral.

INDEFINITE INTEGRALS:

Some important formulae:


n 1
x
n
1.  x dx  n 1  c ;n  
1




n
2.  (ax b) dx  (ax b) n 1  c ;n 
1

(n 1)a


3.  sinx dx   cosx c, sin(ax)dx   cos(ax)  c
a



4.  cosx dx sinx c, cos(ax)dx  sin(ax)  c
a