Page 6 - LESSON NOTES

Eccentricity: The eccentricity of an ellipse is the ratio of the distances from the centre of the
ellipse to one of the foci and to one of the vertices of the ellipse (eccentricity is denoted by e)
c
i.e., e =
a

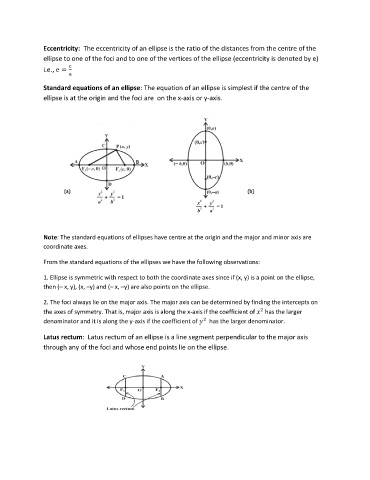
Standard equations of an ellipse: The equation of an ellipse is simplest if the centre of the
ellipse is at the origin and the foci are on the x-axis or y-axis.

Note: The standard equations of ellipses have centre at the origin and the major and minor axis are
coordinate axes.

From the standard equations of the ellipses we have the following observations:

1. Ellipse is symmetric with respect to both the coordinate axes since if (x, y) is a point on the ellipse,
then (– x, y), (x, –y) and (– x, –y) are also points on the ellipse.

2. The foci always lie on the major axis. The major axis can be determined by finding the intercepts on
2
the axes of symmetry. That is, major axis is along the x-axis if the coefficient of has the larger
2
denominator and it is along the y-axis if the coefficient of has the larger denominator.
Latus rectum: Latus rectum of an ellipse is a line segment perpendicular to the major axis
through any of the foci and whose end points lie on the ellipse.