Page 2 - LESSON NOTES

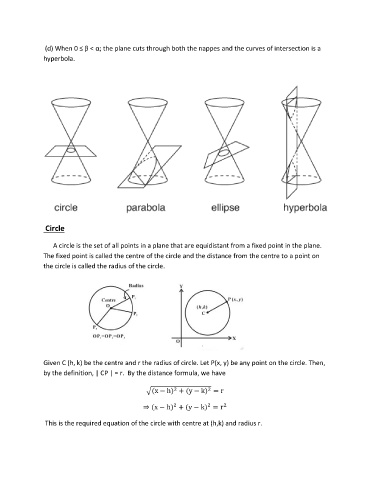
(d) When 0 ≤ β < α; the plane cuts through both the nappes and the curves of intersection is a
hyperbola.

Circle

A circle is the set of all points in a plane that are equidistant from a fixed point in the plane.
The fixed point is called the centre of the circle and the distance from the centre to a point on
the circle is called the radius of the circle.

Given C (h, k) be the centre and r the radius of circle. Let P(x, y) be any point on the circle. Then,
by the definition, | CP | = r. By the distance formula, we have

2
2
√(x − h) + (y − k) = r
2
2
2
⇒ (x − h) + (y − k) = r
This is the required equation of the circle with centre at (h,k) and radius r.