Page 8 - LESSON NOTES

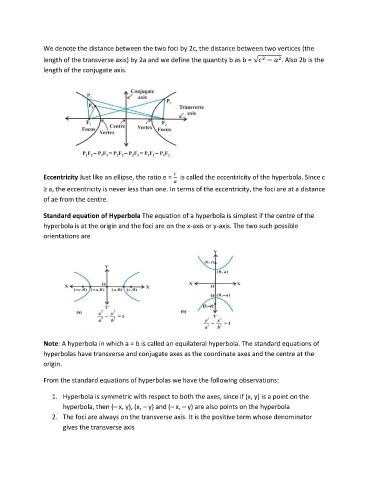
We denote the distance between the two foci by 2c, the distance between two vertices (the

2
2
length of the transverse axis) by 2a and we define the quantity b as b = √ − . Also 2b is the
length of the conjugate axis.

Eccentricity Just like an ellipse, the ratio e = is called the eccentricity of the hyperbola. Since c

≥ a, the eccentricity is never less than one. In terms of the eccentricity, the foci are at a distance
of ae from the centre.

Standard equation of Hyperbola The equation of a hyperbola is simplest if the centre of the
hyperbola is at the origin and the foci are on the x-axis or y-axis. The two such possible
orientations are

Note: A hyperbola in which a = b is called an equilateral hyperbola. The standard equations of
hyperbolas have transverse and conjugate axes as the coordinate axes and the centre at the
origin.

From the standard equations of hyperbolas we have the following observations:

1. Hyperbola is symmetric with respect to both the axes, since if (x, y) is a point on the
hyperbola, then (– x, y), (x, – y) and (– x, – y) are also points on the hyperbola
2. The foci are always on the transverse axis. It is the positive term whose denominator

gives the transverse axis