Page 5 - LESSON NOTES

From the standard equations of the parabolas, we have the following observations:

2
1. Parabola is symmetric with respect to the axis of the parabola. If the equation has a term,
2
then the axis of symmetry is along the x-axis and if the equation has an term, then the axis
of symmetry is along the y-axis.

2. When the axis of symmetry is along the x-axis the parabola opens to the (a) right if the
coefficient of x is positive, (b) left if the coefficient of x is negative.

3. When the axis of symmetry is along the y-axis the parabola opens (c) upwards if the
coefficient of y is positive. (d) Downwards if the coefficient of y is negative.

Ellipse

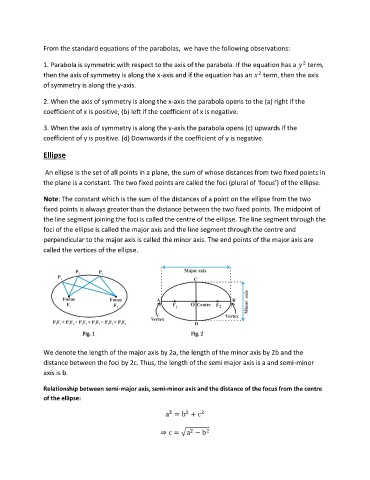
An ellipse is the set of all points in a plane, the sum of whose distances from two fixed points in
the plane is a constant. The two fixed points are called the foci (plural of ‘focus’) of the ellipse.

Note: The constant which is the sum of the distances of a point on the ellipse from the two
fixed points is always greater than the distance between the two fixed points. The midpoint of

the line segment joining the foci is called the centre of the ellipse. The line segment through the
foci of the ellipse is called the major axis and the line segment through the centre and
perpendicular to the major axis is called the minor axis. The end points of the major axis are
called the vertices of the ellipse.

We denote the length of the major axis by 2a, the length of the minor axis by 2b and the
distance between the foci by 2c. Thus, the length of the semi major axis is a and semi-minor
axis is b.

Relationship between semi-major axis, semi-minor axis and the distance of the focus from the centre
of the ellipse:
2

2
a = b + c

2
⇒ c = √a − b