Page 1 - LESSON NOTES

CHAPTER-11 CONIC SECTIONS

LESSON NOTE

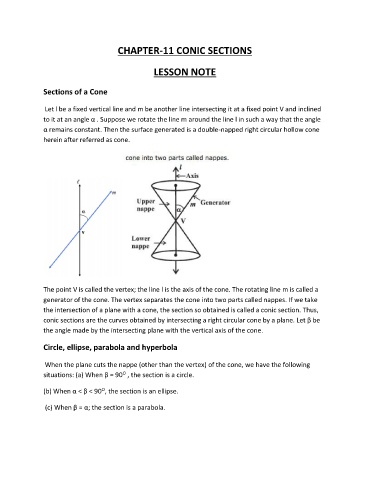
Sections of a Cone

Let l be a fixed vertical line and m be another line intersecting it at a fixed point V and inclined
to it at an angle α . Suppose we rotate the line m around the line l in such a way that the angle
α remains constant. Then the surface generated is a double-napped right circular hollow cone
herein after referred as cone.

The point V is called the vertex; the line l is the axis of the cone. The rotating line m is called a
generator of the cone. The vertex separates the cone into two parts called nappes. If we take
the intersection of a plane with a cone, the section so obtained is called a conic section. Thus,
conic sections are the curves obtained by intersecting a right circular cone by a plane. Let β be

the angle made by the intersecting plane with the vertical axis of the cone.

Circle, ellipse, parabola and hyperbola

When the plane cuts the nappe (other than the vertex) of the cone, we have the following
O
situations: (a) When β = 90 , the section is a circle.

O
(b) When α < β < 90 , the section is an ellipse.
(c) When β = α; the section is a parabola.