Page 13 - NCERT Books

SQUARES AND SQUARE ROOTS 101

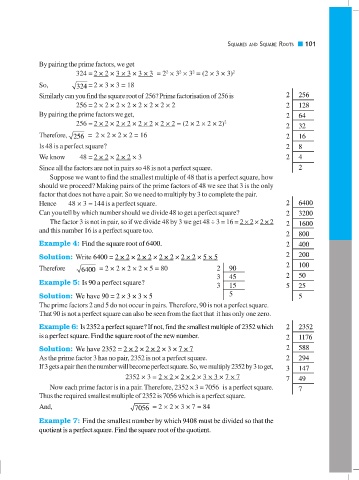
By pairing the prime factors, we get
2
2
2
324 = 2 × 2 × 3 × 3 × 3 × 3 = 2 × 3 × 3 = (2 × 3 × 3) 2
So, 324 = 2 × 3 × 3 = 18
Similarly can you find the square root of 256? Prime factorisation of 256 is 2 256
256 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 2 128
By pairing the prime factors we get, 2 64
256 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 = (2 × 2 × 2 × 2) 2 2 32

Therefore, 256 = 2 × 2 × 2 × 2 = 16 2 16
Is 48 is a perfect square? 2 8
We know 48 = 2 × 2 × 2 × 2 × 3 2 4

Since all the factors are not in pairs so 48 is not a perfect square. 2
Suppose we want to find the smallest multiple of 48 that is a perfect square, how
should we proceed? Making pairs of the prime factors of 48 we see that 3 is the only
factor that does not have a pair. So we need to multiply by 3 to complete the pair.
Hence 48 × 3 = 144 is a perfect square. 2 6400
Can you tell by which number should we divide 48 to get a perfect square? 2 3200
The factor 3 is not in pair, so if we divide 48 by 3 we get 48 ÷ 3 = 16 = 2 × 2 × 2 × 2 2 1600
and this number 16 is a perfect square too.
2 800
Example 4: Find the square root of 6400. 2 400
Solution: Write 6400 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 5 × 5 2 200
2 100
Therefore 6400 = 2 × 2 × 2 × 2 × 5 = 80 2 90
3 45 2 50
Example 5: Is 90 a perfect square?
3 15 5 25
Solution: We have 90 = 2 × 3 × 3 × 5 5 5
The prime factors 2 and 5 do not occur in pairs. Therefore, 90 is not a perfect square.
That 90 is not a perfect square can also be seen from the fact that it has only one zero.

Example 6: Is 2352 a perfect square? If not, find the smallest multiple of 2352 which 2 2352
is a perfect square. Find the square root of the new number. 2 1176

Solution: We have 2352 = 2 × 2 × 2 × 2 × 3 × 7 × 7 2 588
As the prime factor 3 has no pair, 2352 is not a perfect square. 2 294
If 3 gets a pair then the number will become perfect square. So, we multiply 2352 by 3 to get, 3 147

2352 × 3 = 2 × 2 × 2 × 2 × 3 × 3 × 7 × 7 7 49
Now each prime factor is in a pair. Therefore, 2352 × 3 = 7056 is a perfect square. 7
Thus the required smallest multiple of 2352 is 7056 which is a perfect square.
And, 7056 = 2 × 2 × 3 × 7 = 84

Example 7: Find the smallest number by which 9408 must be divided so that the
quotient is a perfect square. Find the square root of the quotient.