Page 16 - NCERT Books

104 MATHEMATICS

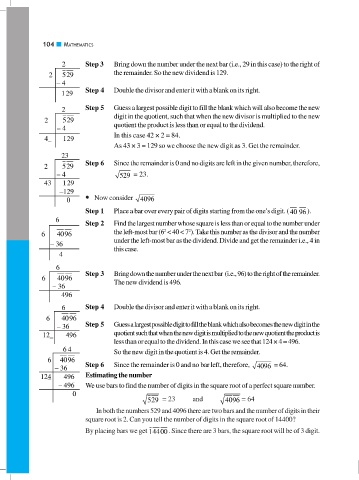
2 Step 3 Bring down the number under the next bar (i.e., 29 in this case) to the right of
2 529 the remainder. So the new dividend is 129.
– 4
Step 4 Double the divisor and enter it with a blank on its right.
129

2 Step 5 Guess a largest possible digit to fill the blank which will also become the new
digit in the quotient, such that when the new divisor is multiplied to the new
2 529
– 4 quotient the product is less than or equal to the dividend.
In this case 42 × 2 = 84.
4_ 129
As 43 × 3 = 129 so we choose the new digit as 3. Get the remainder.
23
Step 6 Since the remainder is 0 and no digits are left in the given number, therefore,
2 529
– 4 529 = 23.
43 129
–129
0 • Now consider 4096
Step 1 Place a bar over every pair of digits starting from the one’s digit. ( 40 96 ).
6
Step 2 Find the largest number whose square is less than or equal to the number under
2
2
6 4096 the left-most bar (6 < 40 < 7 ). Take this number as the divisor and the number
under the left-most bar as the dividend. Divide and get the remainder i.e., 4 in
– 36
this case.
4
6
Step 3 Bring down the number under the next bar (i.e., 96) to the right of the remainder.
6 4096
– 36 The new dividend is 496.
496

6 Step 4 Double the divisor and enter it with a blank on its right.
6 4096
– 36 Step 5 Guess a largest possible digit to fill the blank which also becomes the new digit in the
12_ 496 quotient such that when the new digit is multiplied to the new quotient the product is
less than or equal to the dividend. In this case we see that 124 × 4 = 496.
6 4 So the new digit in the quotient is 4. Get the remainder.
6 4096 Step 6 Since the remainder is 0 and no bar left, therefore, = 64.
– 36 4096
124 496 Estimating the number
– 496 We use bars to find the number of digits in the square root of a perfect square number.
0
529 = 23 and 4096 = 64

In both the numbers 529 and 4096 there are two bars and the number of digits in their
square root is 2. Can you tell the number of digits in the square root of 14400?
By placing bars we get 14400 . Since there are 3 bars, the square root will be of 3 digit.