Page 11 - NCERT Books

SQUARES AND SQUARE ROOTS 99

We know that the area of a square = side 2
If we assume the length of the side to be ‘a’, then 144 = a 2
To find the length of side it is necessary to find a number whose square is 144.
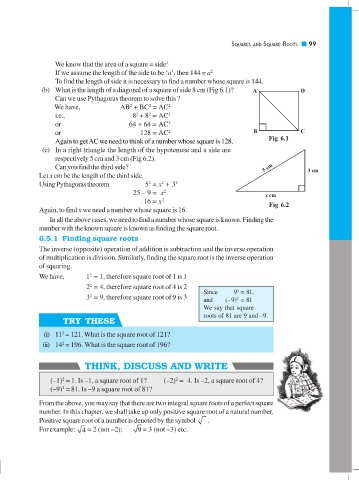
(b) What is the length of a diagonal of a square of side 8 cm (Fig 6.1)?
Can we use Pythagoras theorem to solve this ?
We have, AB + BC = AC 2
2
2
2
2
i.e., 8 + 8 = AC 2
or 64 + 64 = AC 2
or 128 = AC 2
Fig 6.1
Again to get AC we need to think of a number whose square is 128.
(c) In a right triangle the length of the hypotenuse and a side are
respectively 5 cm and 3 cm (Fig 6.2).
Can you find the third side?
Let x cm be the length of the third side.
2
2
Using Pythagoras theorem 5 = x + 3 2
25 – 9 = x 2
16 = x 2
Fig 6.2
Again, to find x we need a number whose square is 16.
In all the above cases, we need to find a number whose square is known. Finding the
number with the known square is known as finding the square root.

6.5.1 Finding square roots
The inverse (opposite) operation of addition is subtraction and the inverse operation
of multiplication is division. Similarly, finding the square root is the inverse operation
of squaring.
2
We have, 1 = 1, therefore square root of 1 is 1
2 = 4, therefore square root of 4 is 2
2
Since 9 = 81,
2
2
3 = 9, therefore square root of 9 is 3 2
and (–9) = 81
We say that square
roots of 81 are 9 and –9.
TRY THESE
2
(i) 11 = 121. What is the square root of 121?
2
(ii) 14 = 196. What is the square root of 196?

THINK, DISCUSS AND WRITE

2
2
(–1) = 1. Is –1, a square root of 1? (–2) = 4. Is –2, a square root of 4?
2
(–9) = 81. Is –9 a square root of 81?
From the above, you may say that there are two integral square roots of a perfect square
number. In this chapter, we shall take up only positive square root of a natural number.
Positive square root of a number is denoted by the symbol .
For example: 4 = 2 (not –2); 9 = 3 (not –3) etc.