Page 6 - Lesson note 5 Angle subtended by arc Ch.- 10 Circle

AOB = 2 APB
AOB = 2 × 50⁰ = 100⁰
Again, OA = OB [Radius of circle]
Then OAB = OBA [Angles opposite to equal sides]
Let OAB = m
In ΔOAB,
By angle sum property: OAB+ OBA+ AOB=180⁰
=> m + m + 100⁰ = 180⁰
=> 2m = 180⁰– 100⁰ = 80⁰
=
=> m = 40⁰

0
OAB = OBA = 40

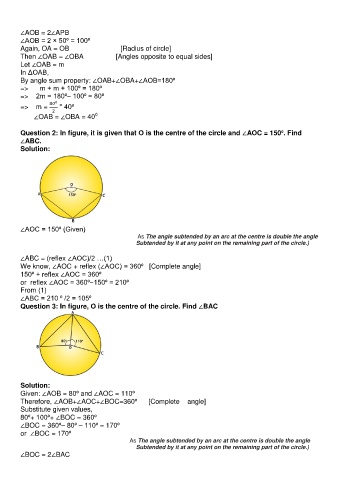
Question 2: In figure, it is given that O is the centre of the circle and AOC = 150⁰. Find
ABC.
Solution:

AOC = 150⁰ (Given)
As The angle subtended by an arc at the centre is double the angle
Subtended by it at any point on the remaining part of the circle.)

ABC = (reflex AOC)/2 …(1)
We know, AOC + reflex ( AOC) = 360⁰ [Complete angle]
150⁰ + reflex AOC = 360⁰
or reflex AOC = 360⁰−150⁰ = 210⁰
From (1)
ABC = 210 ⁰ /2 = 105⁰
Question 3: In figure, O is the centre of the circle. Find BAC

Solution:
Given: AOB = 80⁰ and AOC = 110⁰
Therefore, AOB+ AOC+ BOC=360⁰ [Complete angle]
Substitute given values,
80⁰+ 100⁰+ BOC = 360⁰
BOC = 360⁰– 80⁰ – 110⁰ = 170⁰
or BOC = 170⁰
As The angle subtended by an arc at the centre is double the angle
Subtended by it at any point on the remaining part of the circle.)
BOC = 2 BAC