Page 2 - Lesson note 5 Angle subtended by arc Ch.- 10 Circle

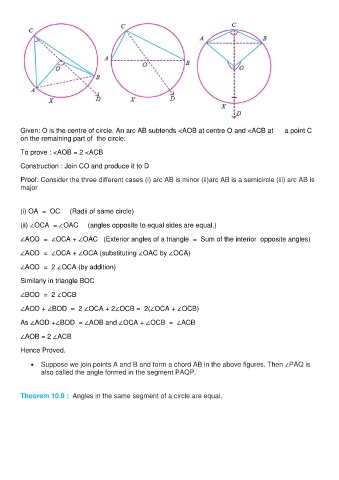
Given: O is the centre of circle. An arc AB subtends <AOB at centre O and <ACB at a point C
on the remaining part of the circle.
To prove : <AOB = 2 <ACB

Construction : Join CO and produce it to D
Proof: Consider the three different cases (i) arc AB is minor (ii)arc AB is a semicircle (iii) arc AB is
major.

(i) OA = OC (Radii of same circle)

(ii) OCA = OAC (angles opposite to equal sides are equal.)

AOD = OCA + OAC (Exterior angles of a triangle = Sum of the interior opposite angles)

AOD = OCA + OCA (substituting OAC by OCA)

AOD = 2 OCA (by addition)

Similarly in triangle BOC

BOD = 2 OCB

AOD + BOD = 2 OCA + 2 OCB = 2( OCA + OCB)

As AOD + BOD = AOB and OCA + OCB = ACB

AOB = 2 ACB

Hence Proved.

 Suppose we join points A and B and form a chord AB in the above figures. Then PAQ is
also called the angle formed in the segment PAQP.

Theorem 10.9 : Angles in the same segment of a circle are equal.