Page 3 - Lesson note-2 Angle subtended by Chord,Ch.- 10 Circle

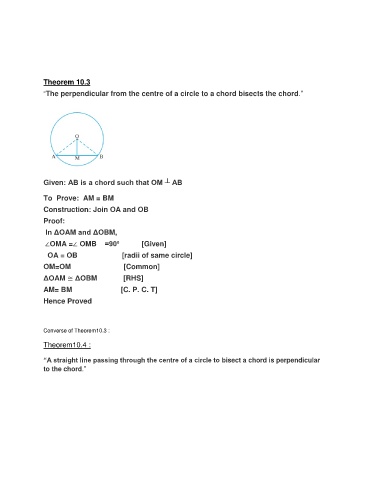
Theorem 10.3
“The perpendicular from the centre of a circle to a chord bisects the chord.”

Given: AB is a chord such that OM ┴ AB

To Prove: AM = BM
Construction: Join OA and OB

Proof:
In ΔOAM and ΔOBM,

∠OMA =∠ OMB =90⁰ [Given]
OA = OB [radii of same circle]
OM=OM [Common]
ΔOAM ≅ ΔOBM [RHS]

AM= BM [C. P. C. T]
Hence Proved

Converse of Theorem10.3 :
Theorem10.4 :

“A straight line passing through the centre of a circle to bisect a chord is perpendicular
to the chord.”