Page 2 - Lesson note-2 Angle subtended by Chord,Ch.- 10 Circle
P. 2
AB = CD [given]
ΔAOB ≅ ΔCOD [SSS]
∠AOB = ∠COD [C. P. C. T]
Hence Proved.
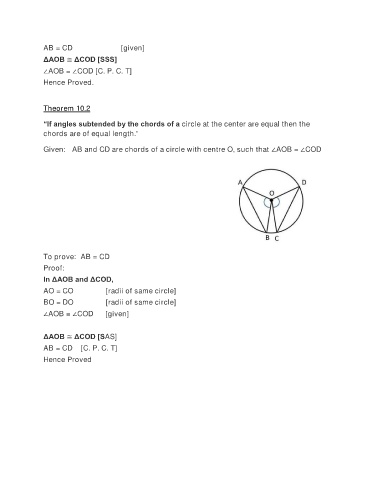
Theorem 10.2
“If angles subtended by the chords of a circle at the center are equal then the
chords are of equal length.”
Given: AB and CD are chords of a circle with centre O, such that ∠AOB = ∠COD
To prove: AB = CD
Proof:
In ΔAOB and ΔCOD,
AO = CO [radii of same circle]
BO = DO [radii of same circle]
∠AOB = ∠COD [given]
ΔAOB ≅ ΔCOD [SAS]
AB = CD [C. P. C. T]
Hence Proved