Page 2 - Lesson note-7 Extra Questions Ch.10 Circle

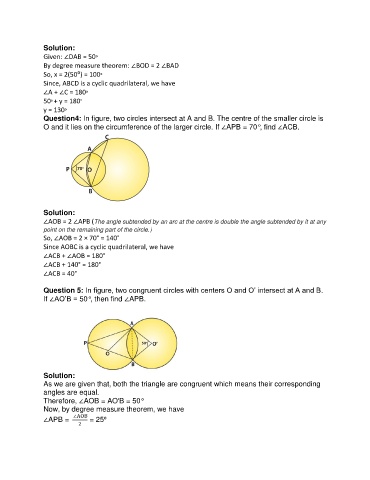
Solution:
Given: ∠DAB = 50⁰
By degree measure theorem: ∠BOD = 2 ∠BAD
So, x = 2(50⁰) = 100⁰
Since, ABCD is a cyclic quadrilateral, we have

∠A + ∠C = 180⁰
50⁰ + y = 180 0
y = 130⁰
Question4: In figure, two circles intersect at A and B. The centre of the smaller circle is
O and it lies on the circumference of the larger circle. If ∠APB = 70°, find ∠ACB.

Solution:
∠AOB = 2 ∠APB (The angle subtended by an arc at the centre is double the angle subtended by it at any
point on the remaining part of the circle.)
So, ∠AOB = 2 × 70° = 140°
Since AOBC is a cyclic quadrilateral, we have
∠ACB + ∠AOB = 180°
∠ACB + 140° = 180°
∠ACB = 40°

Question 5: In figure, two congruent circles with centers O and O’ intersect at A and B.
If ∠AO’B = 50°, then find ∠APB.

Solution:
As we are given that, both the triangle are congruent which means their corresponding
angles are equal.
Therefore, ∠AOB = AO'B = 50°
Now, by degree measure theorem, we have
∠
∠APB = = 25⁰