Page 4 - Lesson Notes-Tangents Ch-10 (Circles)

= AP + AQ + (PX + QX)
We know that
The two tangents drawn from external point to the circle are equal in length from point A,
AB = AC = 5 cm
From point P, PX = PB

From point Q, QX = QC
Perimeter (P) = AP + AQ + (PB + QC)
= (AP + PB) + (AQ + QC)
= AB + AC = 5 + 5

= 10 cm.

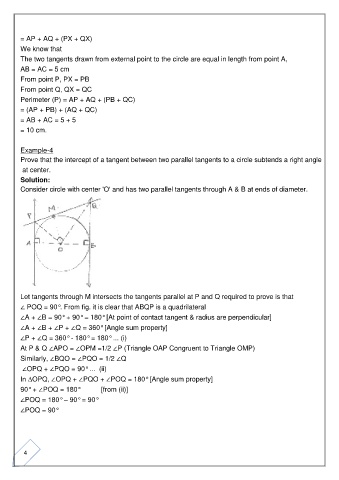
Example-4
Prove that the intercept of a tangent between two parallel tangents to a circle subtends a right angle
at center.

Solution:
Consider circle with center 'O' and has two parallel tangents through A & B at ends of diameter.

Let tangents through M intersects the tangents parallel at P and Q required to prove is that
∠ POQ = 90°. From fig. it is clear that ABQP is a quadrilateral

∠A + ∠B = 90° + 90° = 180° [At point of contact tangent & radius are perpendicular]
∠A + ∠B + ∠P + ∠Q = 360° [Angle sum property]
∠P + ∠Q = 360° - 180° = 180° ... (i)

At P & Q ∠APO = ∠OPM =1/2 ∠P (Triangle OAP Congruent to Triangle OMP)
Similarly, ∠BQO = ∠PQO = 1/2 ∠Q
∠OPQ + ∠PQO = 90° ... (ii)
In ∆OPQ, ∠OPQ + ∠PQO + ∠POQ = 180° [Angle sum property]
90° + ∠POQ = 180° [from (ii)]

∠POQ = 180° – 90° = 90°
∠POQ = 90°

4