Page 3 - Lesson Notes-Tangents Ch-10 (Circles)

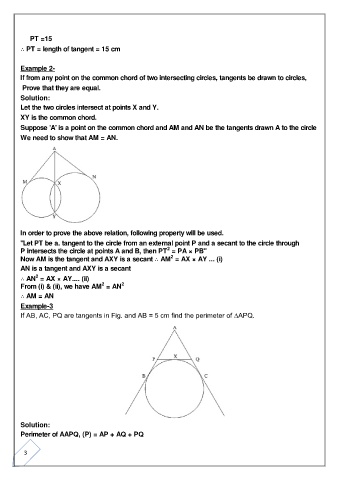
PT =15
∴ PT = length of tangent = 15 cm

Example 2-
If from any point on the common chord of two intersecting circles, tangents be drawn to circles,
Prove that they are equal.

Solution:
Let the two circles intersect at points X and Y.
XY is the common chord.
Suppose 'A' is a point on the common chord and AM and AN be the tangents drawn A to the circle
We need to show that AM = AN.

In order to prove the above relation, following property will be used.
"Let PT be a. tangent to the circle from an external point P and a secant to the circle through
2
P intersects the circle at points A and B, then PT = PA × PB"
2
Now AM is the tangent and AXY is a secant ∴ AM = AX × AY ... (i)
AN is a tangent and AXY is a secant
2
∴ AN = AX × AY.... (ii)
2
2
From (i) & (ii), we have AM = AN
∴ AM = AN
Example-3
If AB, AC, PQ are tangents in Fig. and AB = 5 cm find the perimeter of ∆APQ.

Solution:
Perimeter of AAPQ, (P) = AP + AQ + PQ

3