Page 5 - Lesson Notes-Introduction Ch-10 (Circles)
P. 5
Hence, PAB is an equilateral triangle.
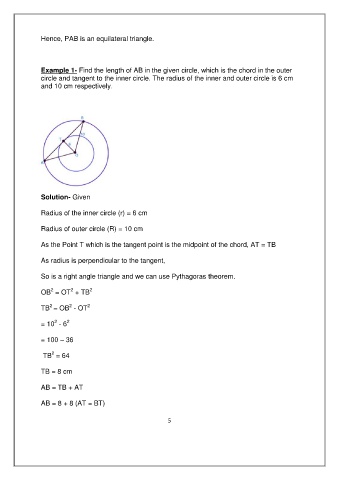
Example 1- Find the length of AB in the given circle, which is the chord in the outer
circle and tangent to the inner circle. The radius of the inner and outer circle is 6 cm
and 10 cm respectively.
Solution- Given
Radius of the inner circle (r) = 6 cm
Radius of outer circle (R) = 10 cm
As the Point T which is the tangent point is the midpoint of the chord, AT = TB
As radius is perpendicular to the tangent,
So is a right angle triangle and we can use Pythagoras theorem.
2
2
2
OB = OT + TB
2
2
2
TB = OB - OT
2
2
= 10 - 6
= 100 – 36
2
TB = 64
TB = 8 cm
AB = TB + AT
AB = 8 + 8 (AT = BT)
5