Page 4 - Lesson Notes-Introduction Ch-10 (Circles)

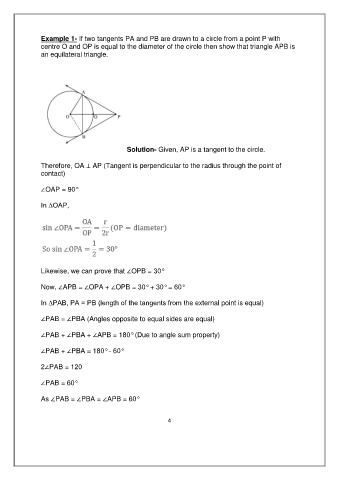
Example 1- If two tangents PA and PB are drawn to a circle from a point P with
centre O and OP is equal to the diameter of the circle then show that triangle APB is
an equilateral triangle.

Solution- Given, AP is a tangent to the circle.

Therefore, OA ⊥ AP (Tangent is perpendicular to the radius through the point of
contact)

∠OAP = 90°

In ∆OAP,

Likewise, we can prove that ∠OPB = 30°

Now, ∠APB = ∠OPA + ∠OPB = 30° + 30° = 60°

In ∆PAB, PA = PB (length of the tangents from the external point is equal)

∠PAB = ∠PBA (Angles opposite to equal sides are equal)

∠PAB + ∠PBA + ∠APB = 180° (Due to angle sum property)

∠PAB + ∠PBA = 180° - 60°

2∠PAB = 120

∠PAB = 60°

As ∠PAB = ∠PBA = ∠APB = 60°

4