Page 4 - Lesson Notes-Word Problems Ch-9 (Applications of Trigonometry)

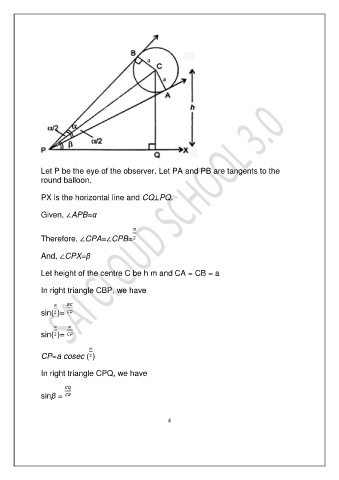
Let P be the eye of the observer. Let PA and PB are tangents to the
round balloon.

PX is the horizontal line and CQ⊥PQ.

Given, ∠APB=α

Therefore, ∠CPA=∠CPB=

And, ∠CPX=β

Let height of the centre C be h m and CA = CB = a

In right triangle CBP, we have

sin( )=

sin( )=

CP=a cosec ( )

In right triangle CPQ, we have

sinβ =

4