Page 3 - Lesson Notes-Word Problems Ch-9 (Applications of Trigonometry)

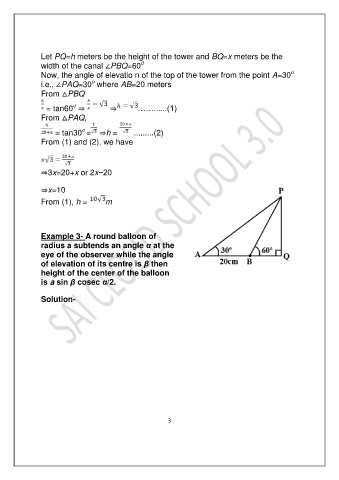
Let PQ=h meters be the height of the tower and BQ=x meters be the
0
width of the canal ∠PBQ=60
o
Now, the angle of elevatio n of the top of the tower from the point A=30
o
i.e., ∠PAQ=30 where AB=20 meters
From △PBQ
o
= tan60 ⇒ ⇒ ……......(1)
From △PAQ,

o
= tan30 = ⇒h = .........(2)
From (1) and (2), we have

⇒3x=20+x or 2x−20

⇒x=10
From (1), h = m

Example 3- A round balloon of
radius a subtends an angle α at the
eye of the observer while the angle
of elevation of its centre is β then
height of the center of the balloon
is a sin β cosec α/2.

Solution-

3