Page 3 - Lesson Notes-Pythagoras Theorem & its Converse-4

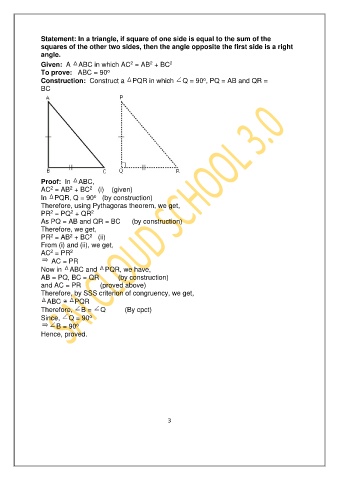
Statement: In a triangle, if square of one side is equal to the sum of the
squares of the other two sides, then the angle opposite the first side is a right
angle.
2
2
Given: A ABC in which AC = AB + BC 2
To prove: ABC = 90 o
o
Construction: Construct a PQR in which Q = 90 , PQ = AB and QR =
BC

Proof: In ABC,
2
2
AC = AB + BC (i) (given)
2
o
In PQR, Q = 90 (by construction)
Therefore, using Pythagoras theorem, we get,
2
2
PR = PQ + QR 2
As PQ = AB and QR = BC (by construction)
Therefore, we get,
PR = AB + BC (ii)
2
2
2
From (i) and (ii), we get,
2
2
AC = PR
AC = PR
Now in ABC and PQR, we have,
AB = PQ, BC = QR (by construction)
and AC = PR (proved above)
Therefore, by SSS criterion of congruency, we get,
ABC PQR
Therefore, B = Q (By cpct)
o
Since, Q = 90
B = 90
o
Hence, proved.

3