Page 2 - Lesson Notes-Pythagoras Theorem & its Converse-4

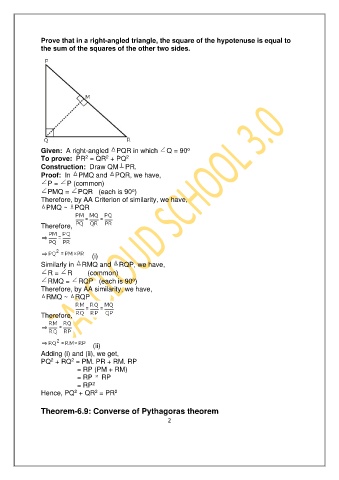
Prove that in a right-angled triangle, the square of the hypotenuse is equal to
the sum of the squares of the other two sides.

o
Given: A right-angled PQR in which Q = 90
2
2
To prove: PR = QR + PQ 2
Construction: Draw QM PR.
Proof: In PMQ and PQR, we have,
P = P (common)
PMQ = PQR (each is 90 )
o
Therefore, by AA Criterion of similarity, we have,
PMQ ~ PQR

Therefore,

(i)
Similarly in RMQ and RQP, we have,
R = R (common)
RMQ = RQP (each is 90 )
o
Therefore, by AA similarity, we have,
RMQ ~ RQP

Therefore,

(ii)
Adding (i) and (ii), we get,
2
PQ + RQ = PM. PR + RM. RP
2
= RP (PM + RM)
= RP RP
= RP 2
2
Hence, PQ + QR = PR
2
2

Theorem-6.9: Converse of Pythagoras theorem
2