Page 1 - Lesson Notes-Pythagoras Theorem & its Converse-4
P. 1
SAI International School
CLASS - X
Mathematics
CHAPTER-6: Triangles-4 Lesson Notes-4
SUBTOPIC : Pythagoras Theorem & its Converse
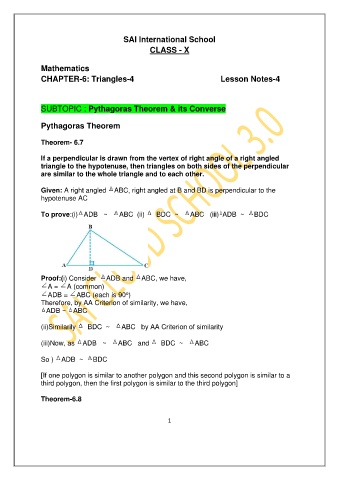
Pythagoras Theorem
Theorem- 6.7
If a perpendicular is drawn from the vertex of right angle of a right angled
triangle to the hypotenuse, then triangles on both sides of the perpendicular
are similar to the whole triangle and to each other.
Given: A right angled ABC, right angled at B and BD is perpendicular to the
hypotenuse AC
To prove:(i) ADB ~ ABC (ii) BDC ~ ABC (iii) ADB ~ BDC
Proof:(i) Consider ADB and ABC, we have,
A = A (common)
ADB = ABC (each is 90 )
o
Therefore, by AA Criterion of similarity, we have,
ADB ~ ABC
(ii)Similarily BDC ~ ABC by AA Criterion of similarity
(iii)Now, as ADB ~ ABC and BDC ~ ABC
So ) ADB ~ BDC
[If one polygon is similar to another polygon and this second polygon is similar to a
third polygon, then the first polygon is similar to the third polygon]
Theorem-6.8
1