Page 3 - Lesson Notes-Relationhip between Zeroes and coefficients Ch-2 (Polynomals)

2
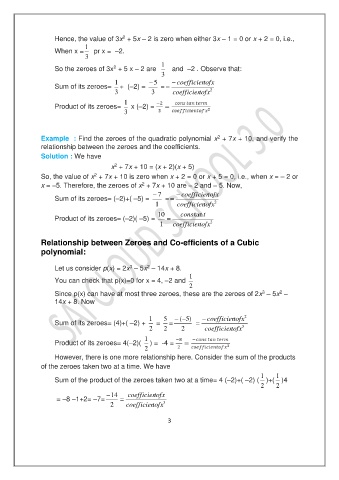
Hence, the value of 3x + 5x – 2 is zero when either 3x – 1 = 0 or x + 2 = 0, i.e.,
1
When x = pr x = –2.
3
1
2
So the zeroes of 3x + 5 x – 2 are and –2 . Observe that:
3
1 − 5 − coefficien tofx
Sum of its zeroes= + (–2) = ==
3 3 coefficien tofx 2

1
Product of its zeroes= x (–2) =
3

2
Example : Find the zeroes of the quadratic polynomial x + 7x + 10, and verify the
relationship between the zeroes and the coefficients.

Solution : We have

x + 7x + 10 = (x + 2)(x + 5)
2

2
So, the value of x + 7x + 10 is zero when x + 2 = 0 or x + 5 = 0, i.e., when x = – 2 or
2
x = –5. Therefore, the zeroes of x + 7x + 10 are – 2 and – 5. Now,
− 7 − coefficien tofx
Sum of its zeroes= (–2)+( –5) = ==
1 coefficien tofx 2
10 cons tant
Product of its zeroes= (–2)( –5) = =
1 coefficien tofx 2

Relationship between Zeroes and Co-efficients of a Cubic
polynomial:

Let us consider p(x) = 2x – 5x – 14x + 8.
3
2
1
You can check that p(x)=0 for x = 4, –2 and
2
Since p(x) can have at most three zeroes, these are the zeroes of 2x – 5x –
3
2
14x + 8. Now

1 5 − (− ) 5 − coefficien tofx 2
Sum of its zeroes= (4)+( –2) + = = =
2 2 2 coefficien tofx 3
1
Product of its zeroes= 4(–2)( ) = -4 =
2
However, there is one more relationship here. Consider the sum of the products

of the zeroes taken two at a time. We have
1 1
Sum of the product of the zeroes taken two at a time= 4 (–2)+( –2) ( )+( )4
2 2
− 14 coefficien tofx
= –8 –1+2= –7= =
2 coefficien tofx 3

3