Page 2 - Lesson Notes-Relationhip between Zeroes and coefficients Ch-2 (Polynomals)

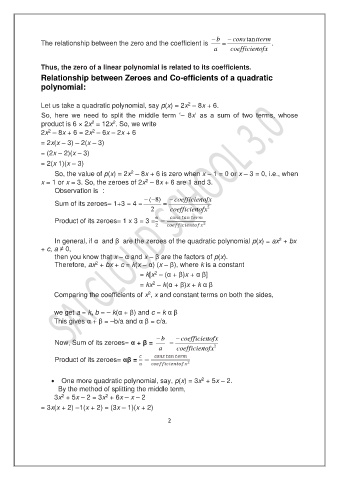
− b − constan tterm
The relationship between the zero and the coefficient is = .
a coefficien tofx

Thus, the zero of a linear polynomial is related to its coefficients.
Relationship between Zeroes and Co-efficients of a quadratic
polynomial:

2
Let us take a quadratic polynomial, say p(x) = 2x – 8x + 6.
So, here we need to split the middle term ‘– 8x’ as a sum of two terms, whose
product is 6 × 2x = 12x . So, we write
2
2
2x – 8x + 6 = 2x – 6x – 2x + 6
2
2
= 2x(x – 3) – 2(x – 3)
= (2x – 2)(x – 3)
= 2(x 1)(x – 3)
2
So, the value of p(x) = 2x – 8x + 6 is zero when x – 1 = 0 or x – 3 = 0, i.e., when
2
x = 1 or x = 3. So, the zeroes of 2x – 8x + 6 are 1 and 3.
Observation is :
− (− ) 8 − coefficien tofx
Sum of its zeroes= 1+3 = 4 = =
2 coefficien tofx 2

Product of its zeroes= 1 x 3 = 3 =

2
In general, if α and β are the zeroes of the quadratic polynomial p(x) = ax + bx
+ c, a ≠ 0,
then you know that x – α and x – β are the factors of p(x).
2
Therefore, ax + bx + c = k(x – α) (x – β), where k is a constant
= k[x – (α + β)x + α β]
2

2
= kx – k(α + β)x + k α β
Comparing the coefficients of x , x and constant terms on both the sides,
2

we get a = k, b = – k(α + β) and c = k α β
This gives α + β = –b/a and α β = c/a.

− b − coefficien tofx
Now, Sum of its zeroes= α + β = =
a coefficien tofx 2
Product of its zeroes= αβ =

2
• One more quadratic polynomial, say, p(x) = 3x + 5x – 2.
By the method of splitting the middle term,
2
2
3x + 5x – 2 = 3x + 6x – x – 2
= 3x(x + 2) –1(x + 2) = (3x – 1)(x + 2)

2