Page 1 - NCERT Books

SQUARES AND SQUARE ROOTS 89

CHAPTER
Squares and Square

Roots 6

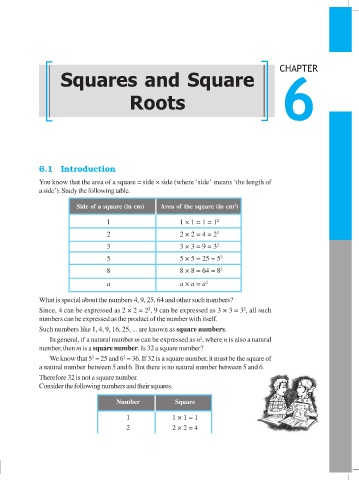
6.1 Introduction

You know that the area of a square = side × side (where ‘side’ means ‘the length of
a side’). Study the following table.

2
Side of a square (in cm) Area of the square (in cm )
1 1 × 1 = 1 = 1 2

2 2 × 2 = 4 = 2 2
3 3 × 3 = 9 = 3 2

5 5 × 5 = 25 = 5 2
8 8 × 8 = 64 = 8 2

a a × a = a 2

What is special about the numbers 4, 9, 25, 64 and other such numbers?
2
2
Since, 4 can be expressed as 2 × 2 = 2 , 9 can be expressed as 3 × 3 = 3 , all such
numbers can be expressed as the product of the number with itself.
Such numbers like 1, 4, 9, 16, 25, ... are known as square numbers.
2
In general, if a natural number m can be expressed as n , where n is also a natural
number, then m is a square number. Is 32 a square number?
2
2
We know that 5 = 25 and 6 = 36. If 32 is a square number, it must be the square of
a natural number between 5 and 6. But there is no natural number between 5 and 6.
Therefore 32 is not a square number.
Consider the following numbers and their squares.

Number Square

1 1 × 1 = 1
2 2 × 2 = 4