Page 2 - Lesson Notes-Ch.13 SA and Volumes(Cone)

Volume of a Right Circular Cone

The volume of a cone defines the space or the capacity of the cone. A cone is a
three-dimensional geometric shape having a circular base that becomes narrower
from a flat base to a point called vertex.

Derivation of Cone Volume

You can think of a cone as a triangle which is being rotated about one of its vertices.
Now, think of a scenario where we need to calculate the amount of water that can be
accommodated in a conical flask. In other words, we mean to calculate the capacity
of this flask. The capacity of a conical flask is basically equal to the volume of the
cone involved. Thus, the volume of a three-dimensional shape is equal to the
amount of space occupied by that shape. Let us perform an activity to calculate the
volume of a cone.

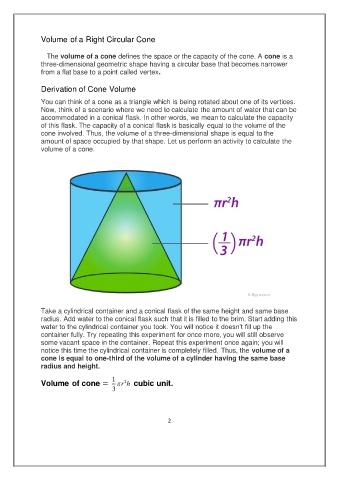
Take a cylindrical container and a conical flask of the same height and same base
radius. Add water to the conical flask such that it is filled to the brim. Start adding this
water to the cylindrical container you took. You will notice it doesn’t fill up the
container fully. Try repeating this experiment for once more, you will still observe
some vacant space in the container. Repeat this experiment once again; you will
notice this time the cylindrical container is completely filled. Thus, the volume of a
cone is equal to one-third of the volume of a cylinder having the same base
radius and height.

1
2
Volume of cone =  r h cubic unit.
3

2