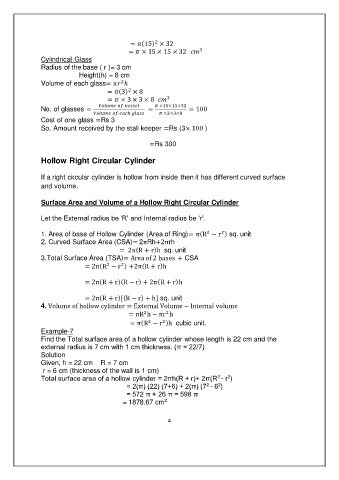
Page 4 - Lesson Notes-2-Ch.13 SA and Volumes(Cylinder)

2
= (15) × 32
= × 15 × 15 × 32
3
Cylindrical Glass
Radius of the base ( r )= 3 cm
Height(h) = 8 cm
2
Volume of each glass= ℎ
2
= (3) × 8
= × 3 × 3 × 8
3
×15×15×32
No. of glasses = = = 100
ℎ ×3×3×8
Cost of one glass =Rs 3
So, Amount received by the stall keeper =Rs (3× 100 )

=Rs 300

Hollow Right Circular Cylinder

If a right circular cylinder is hollow from inside then it has different curved surface
and volume.

Surface Area and Volume of a Hollow Right Circular Cylinder

Let the External radius be ‘R’ and Internal radius be ‘r’.

1. Area of base of Hollow Cylinder (Area of Ring)= π(R − r ) sq. unit
2
2
2. Curved Surface Area (CSA)= 2πRh+2πrh
= 2π(R + r)h sq. unit
3.Total Surface Area (TSA)= Area of 2 bases + CSA
2
2
= 2π(R − r ) +2π(R + r)h

= 2π(R + r)(R − r) + 2π(R + r)h

= 2π(R + r)[(R − r) + h] sq. unit
4. Volume of hollow cylinder = External Volume − Internal volume
= πR h − πr h
2
2
= π(R − r )h cubic unit.
2
2
Example-7
Find the Total surface area of a hollow cylinder whose length is 22 cm and the
external radius is 7 cm with 1 cm thickness. (π = 22/7)
Solution
Given, h = 22 cm R = 7 cm
r = 6 cm (thickness of the wall is 1 cm)
2
2
Total surface area of a hollow cylinder = 2πh(R + r)+ 2π(R - r )
2
2
= 2(π) (22) (7+6) + 2(π) (7 - 6 )
= 572 π + 26 π = 598 π
2
= 1878.67 cm
4