Page 2 - Home Assignment-7 Extra Questions Ch.10 Circle

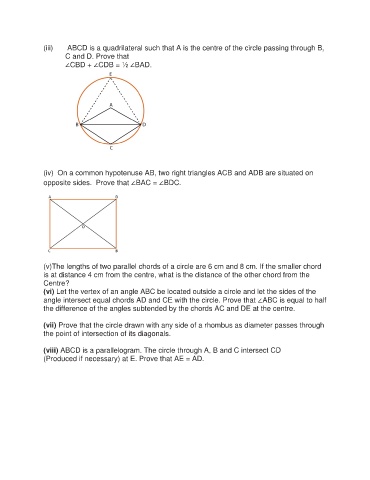
(iii) ABCD is a quadrilateral such that A is the centre of the circle passing through B,
C and D. Prove that
∠CBD + ∠CDB = ½ ∠BAD.

(iv) On a common hypotenuse AB, two right triangles ACB and ADB are situated on
opposite sides. Prove that ∠BAC = ∠BDC.

(v)The lengths of two parallel chords of a circle are 6 cm and 8 cm. If the smaller chord
is at distance 4 cm from the centre, what is the distance of the other chord from the
Centre?
(vi) Let the vertex of an angle ABC be located outside a circle and let the sides of the
angle intersect equal chords AD and CE with the circle. Prove that ∠ABC is equal to half
the difference of the angles subtended by the chords AC and DE at the centre.

(vii) Prove that the circle drawn with any side of a rhombus as diameter passes through
the point of intersection of its diagonals.

(viii) ABCD is a parallelogram. The circle through A, B and C intersect CD
(Produced if necessary) at E. Prove that AE = AD.