Page 2 - Activity 3 Opposite angles of Cyclic Quadrilateral Ch.10 Circle

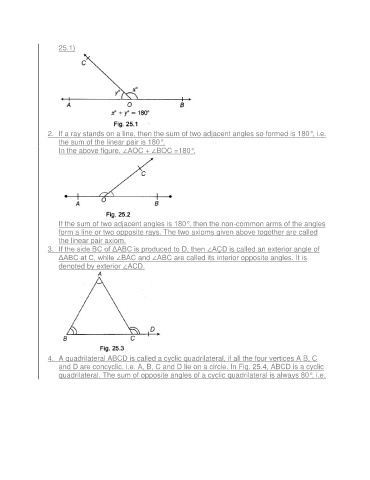
25.1)

2. If a ray stands on a line, then the sum of two adjacent angles so formed is 180°, i.e.
the sum of the linear pair is 180°.
In the above figure, ∠AOC + ∠BOC =180°.

If the sum of two adjacent angles is 180°, then the non-common arms of the angles
form a line or two opposite rays. The two axioms given above together are called
the linear pair axiom.
3. If the side BC of ΔABC is produced to D, then ∠AÇD is called an exterior angle of
ΔABC at C, while ∠BAC and ∠ABC are called its interior opposite angles. It is
denoted by exterior ∠ACD.

4. A quadrilateral ABCD is called a cyclic quadrilateral, if all the four vertices A B, C
and D are concyclic, i.e. A, B, C and D lie on a circle. In Fig. 25.4, ABCD is a cyclic
quadrilateral. The sum of opposite angles of a cyclic quadrilateral is always 80°, i.e.