Page 5 - Lesson note-6 Cyclic Quadrilateral Ch. 10 circle

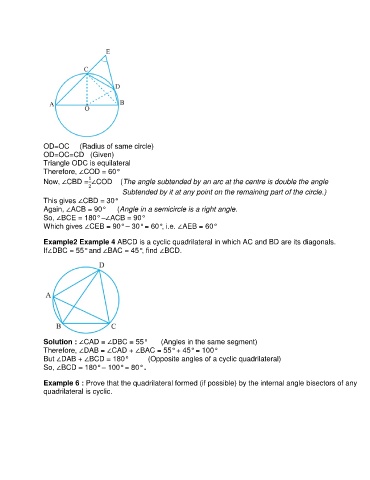
OD=OC (Radius of same circle)
OD=OC=CD (Given)
Triangle ODC is equilateral
Therefore, ∠COD = 60°

Now, ∠CBD = ∠COD (The angle subtended by an arc at the centre is double the angle

Subtended by it at any point on the remaining part of the circle.)
This gives ∠CBD = 30°
Again, ∠ACB = 90° (Angle in a semicircle is a right angle.
So, ∠BCE = 180° –∠ACB = 90°
Which gives ∠CEB = 90° – 30° = 60°, i.e. ∠AEB = 60°

Example2 Example 4 ABCD is a cyclic quadrilateral in which AC and BD are its diagonals.
If∠DBC = 55° and ∠BAC = 45°, find ∠BCD.

Solution : ∠CAD = ∠DBC = 55° (Angles in the same segment)
Therefore, ∠DAB = ∠CAD + ∠BAC = 55° + 45° = 100°
But ∠DAB + ∠BCD = 180° (Opposite angles of a cyclic quadrilateral)
So, ∠BCD = 180° – 100° = 80° .

Example 6 : Prove that the quadrilateral formed (if possible) by the internal angle bisectors of any
quadrilateral is cyclic.