Page 2 - Lesson note 4 Equal Chords-equidistant- circle Ch.- 10 Circle
P. 2
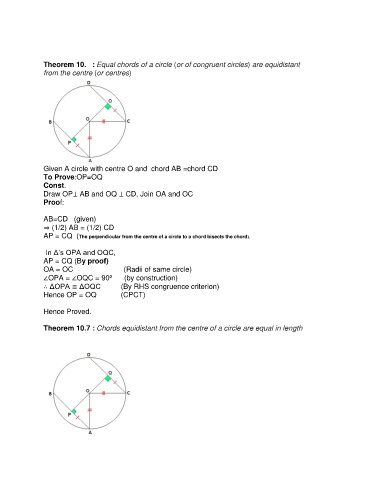
Theorem 10.6 : Equal chords of a circle (or of congruent circles) are equidistant
from the centre (or centres)
Given A circle with centre O and chord AB =chord CD
To Prove:OP=OQ
Const.
Draw OP⊥ AB and OQ ⊥ CD, Join OA and OC
Proof:
AB=CD (given)
⇒ (1/2) AB = (1/2) CD
AP = CQ (The perpendicular from the centre of a circle to a chord bisects the chord).
In Δ’s OPA and OQC,
AP = CQ (By proof)
OA = OC (Radii of same circle)
∠OPA = ∠OQC = 90⁰ (by construction)
∴ ΔOPA ≅ ΔOQC (By RHS congruence criterion)
Hence OP = OQ (CPCT)
Hence Proved.
Theorem 10.7 : Chords equidistant from the centre of a circle are equal in length