Page 3 - IX Lesson Note- 3 Ttriangles having equal- ch 9 Area of Parallelograms and Triangles
P. 3
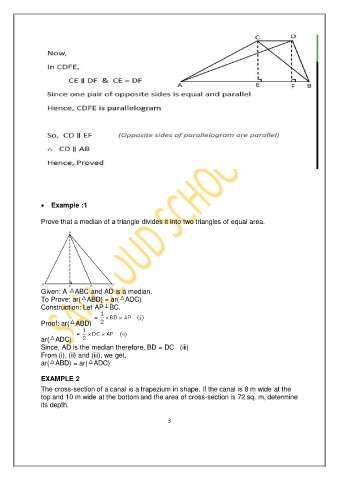
• Example :1
Prove that a median of a triangle divides it into two triangles of equal area.
Given: A ABC and AD is a median.
To Prove: ar( ABD) = ar( ADC)
Construction: Let AP BC.
Proof: ar( ABD)
ar( ADC)
Since, AD is the median therefore, BD = DC (iii)
From (i), (ii) and (iii), we get,
ar( ABD) = ar( ADC).
EXAMPLE 2
The cross-section of a canal is a trapezium in shape. If the canal is 8 m wide at the
top and 10 m wide at the bottom and the area of cross-section is 72 sq. m, determine
its depth.
3