Page 2 - IX Lesson Note -2 triangles on same base-ch 9(Area of Parallelograms and Triangles

We know that the diagonals of a parallelogram divides it into two triangles of equal
area.

Therefore, area( PBC) = area(parallelogram BCQP) (2)

and area( DBC) = area(parallelogram BCAD) (3)

Now, area(parallelogram BCQP) = area(parallelogram BCAD) [from (1)]

area(parallelogram BCAD) = area(parallelogram BCQP)

area( ABC) = area( PBC) [using (2) and (3)]

Hence, area( ABC) = area( PBC)

Example: 1

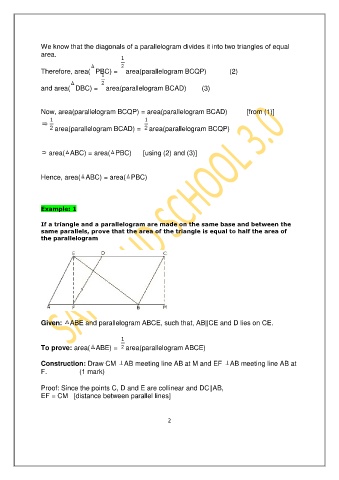
If a triangle and a parallelogram are made on the same base and between the
same parallels, prove that the area of the triangle is equal to half the area of
the parallelogram

Given: ABE and parallelogram ABCE, such that, AB||CE and D lies on CE.

To prove: area( ABE) = area(parallelogram ABCE)

Construction: Draw CM AB meeting line AB at M and EF AB meeting line AB at
F. (1 mark)

Proof: Since the points C, D and E are collinear and DC||AB,
EF = CM [distance between parallel lines]

2