Page 3 - IX Lesson Note 1area and properties- ch 9 Area of Parallelograms and Triangles

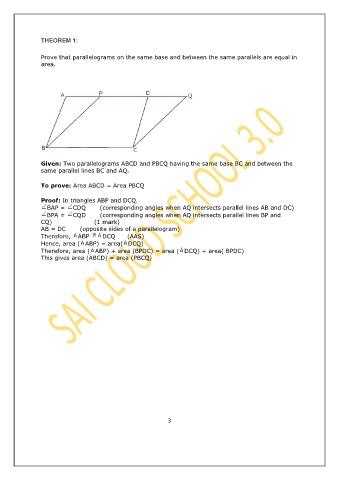
THEOREM 1:

Prove that parallelograms on the same base and between the same parallels are equal in
area.

Given: Two parallelograms ABCD and PBCQ having the same base BC and between the
same parallel lines BC and AQ.

To prove: Area ABCD = Area PBCQ

Proof: In triangles ABP and DCQ.
BAP = CDQ (corresponding angles when AQ intersects parallel lines AB and DC)
BPA = CQD (corresponding angles when AQ intersects parallel lines BP and
CQ) (1 mark)
AB = DC (opposite sides of a parallelogram)
Therefore, ABP DCQ (AAS)
Hence, area ( ABP) = area( DCQ)
Therefore, area ( ABP) + area (BPDC) = area ( DCQ) + area( BPDC)
This gives area (ABCD) = area (PBCQ)

3