Page 5 - Lesson Notes-Euclid Definitions,Axioms and Postulates Ch-5(Introduction to Euclid')

This circle is divided into four parts and

each part is smaller than the whole circle. This shows that the whole

circle will always be greater than any of its parts.

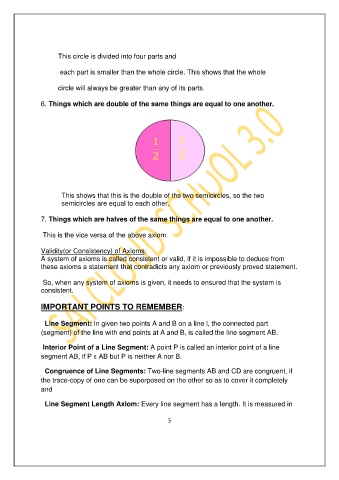
6. Things which are double of the same things are equal to one another.

This shows that this is the double of the two semicircles, so the two
semicircles are equal to each other.

7. Things which are halves of the same things are equal to one another.

This is the vice versa of the above axiom.

Validity(or Consistency) of Axioms:
A system of axioms is called consistent or valid, if it is impossible to deduce from
these axioms a statement that contradicts any axiom or previously proved statement.

So, when any system of axioms is given, it needs to ensured that the system is
consistent.

IMPORTANT POINTS TO REMEMBER:

Line Segment: In given two points A and B on a line l, the connected part
(segment) of the line with end points at A and B, is called the line segment AB.

Interior Point of a Line Segment: A point P is called an interior point of a line
segment AB, if P ε AB but P is neither A nor B.

Congruence of Line Segments: Two-line segments AB and CD are congruent, if
the trace-copy of one can be superposed on the other so as to cover it completely
and

Line Segment Length Axiom: Every line segment has a length. It is measured in

5