Page 2 - ln

 In the common factor method we chose our factor as one term but in this
method common factor can be more than one term.
 Parenthesis helps us in taking group of term as common. So it is important to
give parenthesis in proper way.

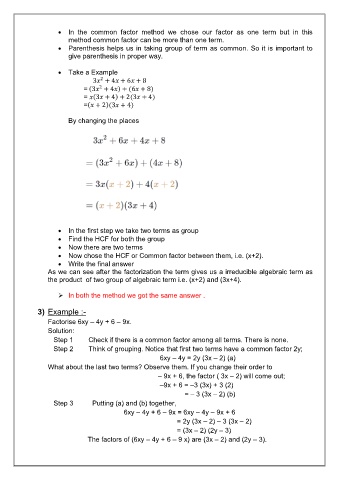
 Take a Example

Ͷ ͸

= Ͷ 㜮 ͸ܙ
= Ͷ 㜮 Ͷܙ
= 㜮 Ͷܙ

By changing the places

 In the first step we take two terms as group
 Find the HCF for both the group
 Now there are two terms
 Now chose the HCF or Common factor between them, i.e. (x+2).
 Write the final answer
As we can see after the factorization the term gives us a irreducible algebraic term as
the product of two group of algebraic term i.e. (x+2) and (3x+4).

 In both the method we got the same answer .

3) Example :-

Factorise 6xy – 4y + 6 – 9x.
Solution:
Step 1 Check if there is a common factor among all terms. There is none.
Step 2 Think of grouping. Notice that first two terms have a common factor 2y;
6xy – 4y = 2y (3x – 2) (a)
What about the last two terms? Observe them. If you change their order to
– 9x + 6, the factor ( 3x – 2) will come out;
–9x + 6 = –3 (3x) + 3 (2)
= – 3 (3x – 2) (b)
Step 3 Putting (a) and (b) together,
6xy – 4y + 6 – 9x = 6xy – 4y – 9x + 6
= 2y (3x – 2) – 3 (3x – 2)
= (3x – 2) (2y – 3)
The factors of (6xy – 4y + 6 – 9 x) are (3x – 2) and (2y – 3).