Page 1 - ln
P. 1
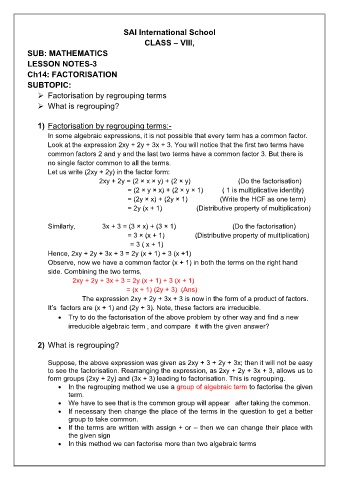
SAI International School
CLASS – VIII,
SUB: MATHEMATICS
LESSON NOTES-3
Ch14: FACTORISATION
SUBTOPIC:
Factorisation by regrouping terms
What is regrouping?
1) Factorisation by regrouping terms:-
In some algebraic expressions, it is not possible that every term has a common factor.
Look at the expression 2xy + 2y + 3x + 3. You will notice that the first two terms have
common factors 2 and y and the last two terms have a common factor 3. But there is
no single factor common to all the terms.
Let us write (2xy + 2y) in the factor form:
2xy + 2y = (2 × x × y) + (2 × y) (Do the factorisation)
= (2 × y × x) + (2 × y × 1) ( 1 is multiplicative identity)
= (2y × x) + (2y × 1) (Write the HCF as one term)
= 2y (x + 1) (Distributive property of multiplication)
Similarly, 3x + 3 = (3 × x) + (3 × 1) (Do the factorisation)
= 3 × (x + 1) (Distributive property of multiplication)
= 3 ( x + 1)
Hence, 2xy + 2y + 3x + 3 = 2y (x + 1) + 3 (x +1)
Observe, now we have a common factor (x + 1) in both the terms on the right hand
side. Combining the two terms,
2xy + 2y + 3x + 3 = 2y (x + 1) + 3 (x + 1)
= (x + 1) (2y + 3) (Ans)
The expression 2xy + 2y + 3x + 3 is now in the form of a product of factors.
It’s factors are (x + 1) and (2y + 3). Note, these factors are irreducible.
Try to do the factorisation of the above problem by other way and find a new
irreducible algebraic term , and compare it with the given answer?
2) What is regrouping?
Suppose, the above expression was given as 2xy + 3 + 2y + 3x; then it will not be easy
to see the factorisation. Rearranging the expression, as 2xy + 2y + 3x + 3, allows us to
form groups (2xy + 2y) and (3x + 3) leading to factorisation. This is regrouping.
In the regrouping method we use a group of algebraic term to factorise the given
term.
We have to see that is the common group will appear after taking the common.
If necessary then change the place of the terms in the question to get a better
group to take common.
If the terms are written with assign + or – then we can change their place with
the given sign
In this method we can factorise more than two algebraic terms