Page 2 - ln

STEP 1 :Divide the given natural number by its smallest prime factor

STEP 2 :Divide the quotient obtained in step 1, by its smallest prime factor.
Go on dividing each of the subsequent quotients by their smallest prime factors, till the
last quotient is 1.

STEP 3 :Express the given natural number as the product of all these factors.
This becomes the Prime Factorization of the natural number.
The steps and the method of presentation will be clear by the following examples.

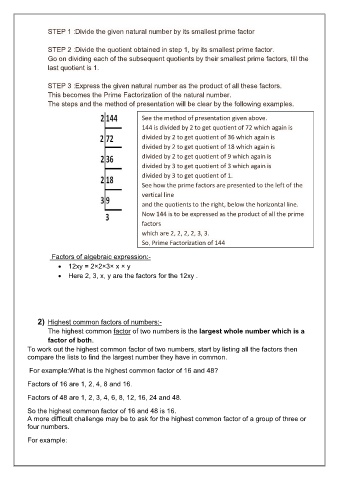
See the method of presentation given above.
144 is divided by 2 to get quotient of 72 which again is
divided by 2 to get quotient of 36 which again is
divided by 2 to get quotient of 18 which again is
divided by 2 to get quotient of 9 which again is
divided by 3 to get quotient of 3 which again is

divided by 3 to get quotient of 1.
See how the prime factors are presented to the left of the
vertical line
and the quotients to the right, below the horizontal line.
Now 144 is to be expressed as the product of all the prime
factors
which are 2, 2, 2, 2, 3, 3.
So, Prime Factorization of 144

Factors of algebraic expression:-
 12xy = 2×2×3× x × y

 Here 2, 3, x, y are the factors for the 12xy .

2) Highest common factors of numbers:-
The highest common factor of two numbers is the largest whole number which is a
factor of both.
To work out the highest common factor of two numbers, start by listing all the factors then
compare the lists to find the largest number they have in common.

For example:What is the highest common factor of 16 and 48?

Factors of 16 are 1, 2, 4, 8 and 16.

Factors of 48 are 1, 2, 3, 4, 6, 8, 12, 16, 24 and 48.

So the highest common factor of 16 and 48 is 16.
A more difficult challenge may be to ask for the highest common factor of a group of three or
four numbers.

For example: