Page 2 - MM1
P. 2
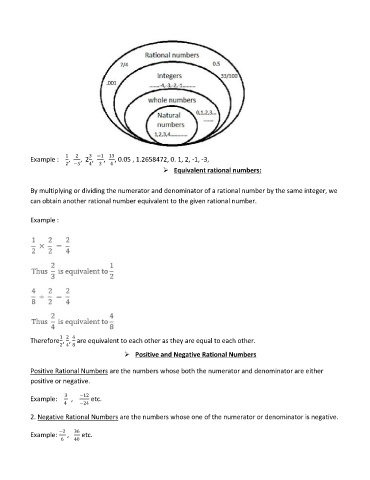
1 2 3 −1 13
Example : , , 2 , , , 0.05 , 1.2658472, 0. 1, 2, -1, -3,
2 −5 4 3 4
Equivalent rational numbers:
By multiplying or dividing the numerator and denominator of a rational number by the same integer, we
can obtain another rational number equivalent to the given rational number.
Example :
1 2 4
Therefore , , are equivalent to each other as they are equal to each other.
2 4 8
Positive and Negative Rational Numbers
Positive Rational Numbers are the numbers whose both the numerator and denominator are either
positive or negative.
3 −12
Example: , etc.
4 −24
2. Negative Rational Numbers are the numbers whose one of the numerator or denominator is negative.
−2 36
Example: , etc.
6 40