Page 2 - LESSION NOTES CH-8 PDF
P. 2
A=∫ =∫ dx=∫ ( )dx
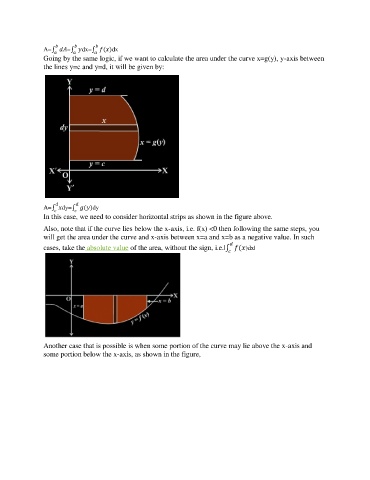
Going by the same logic, if we want to calculate the area under the curve x=g(y), y-axis between
the lines y=c and y=d, it will be given by:
A=∫ dy=∫ ( )dy
In this case, we need to consider horizontal strips as shown in the figure above.
Also, note that if the curve lies below the x-axis, i.e. f(x) <0 then following the same steps, you
will get the area under the curve and x-axis between x=a and x=b as a negative value. In such
cases, take the absolute value of the area, without the sign, i.e.|∫ ( )dx|
Another case that is possible is when some portion of the curve may lie above the x-axis and
some portion below the x-axis, as shown in the figure,