Page 1 - LESSION NOTES CH-8 PDF
P. 1
LESSION LL
LESSION NOTES
APPLICATION OF INTEGRALS.
CHAPTER -8
In Calculus, we have seen definite integral as a limit of a sum and we know how to evaluate it
using the fundamental theorem of calculus. In the upcoming discussion, we will see an easier
way of finding the area bounded by any curve and x-axis between given coordinates. To
determine the area under the curve we follow the following method.
How to Determine the Area Under the Curve?
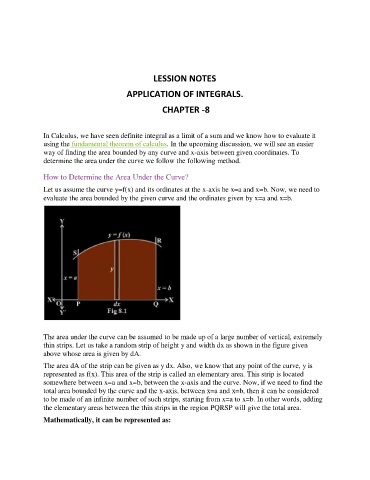
Let us assume the curve y=f(x) and its ordinates at the x-axis be x=a and x=b. Now, we need to
evaluate the area bounded by the given curve and the ordinates given by x=a and x=b.
The area under the curve can be assumed to be made up of a large number of vertical, extremely
thin strips. Let us take a random strip of height y and width dx as shown in the figure given
above whose area is given by dA.
The area dA of the strip can be given as y dx. Also, we know that any point of the curve, y is
represented as f(x). This area of the strip is called an elementary area. This strip is located
somewhere between x=a and x=b, between the x-axis and the curve. Now, if we need to find the
total area bounded by the curve and the x-axis, between x=a and x=b, then it can be considered
to be made of an infinite number of such strips, starting from x=a to x=b. In other words, adding
the elementary areas between the thin strips in the region PQRSP will give the total area.
Mathematically, it can be represented as: