Page 2 - Lesson note-Area of sector,length of Arc

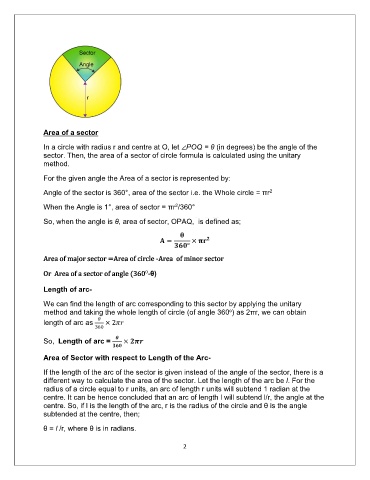
Area of a sector

In a circle with radius r and centre at O, let ∠POQ = θ (in degrees) be the angle of the
sector. Then, the area of a sector of circle formula is calculated using the unitary
method.

For the given angle the Area of a sector is represented by:

Angle of the sector is 360°, area of the sector i.e. the Whole circle = πr 2

When the Angle is 1°, area of sector = πr /360°
2
So, when the angle is θ, area of sector, OPAQ, is defined as;

t t

Area of major sector tArea of circle -Area of minor sector
Or Area of a sector of angle (360 -θ)
0

Length of arc-

We can find the length of arc corresponding to this sector by applying the unitary
method and taking the whole length of circle (of angle 360 ) as 2πr, we can obtain
o
length of arc as
360
So, Length of arc =

Area of Sector with respect to Length of the Arc-

If the length of the arc of the sector is given instead of the angle of the sector, there is a
different way to calculate the area of the sector. Let the length of the arc be l. For the
radius of a circle equal to r units, an arc of length r units will subtend 1 radian at the
centre. It can be hence concluded that an arc of length l will subtend l/r, the angle at the
centre. So, if l is the length of the arc, r is the radius of the circle and θ is the angle
subtended at the centre, then;

θ = l /r, where θ is in radians.

2