Page 2 - Lesson Note 2- ch 11 (Contruction of Tangents)

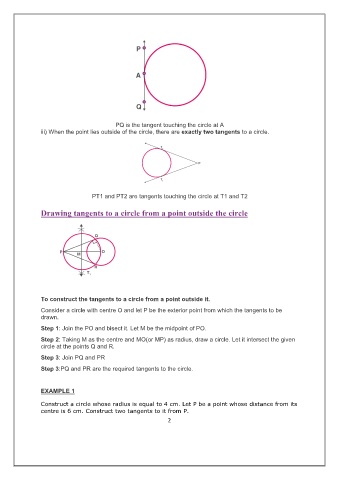
PQ is the tangent touching the circle at A
iii) When the point lies outside of the circle, there are exactly two tangents to a circle.

PT1 and PT2 are tangents touching the circle at T1 and T2

Drawing tangents to a circle from a point outside the circle

To construct the tangents to a circle from a point outside it.
Consider a circle with centre O and let P be the exterior point from which the tangents to be
drawn.
Step 1: Join the PO and bisect it. Let M be the midpoint of PO.

Step 2: Taking M as the centre and MO(or MP) as radius, draw a circle. Let it intersect the given
circle at the points Q and R.

Step 3: Join PQ and PR
Step 3:PQ and PR are the required tangents to the circle.

EXAMPLE 1

Construct a circle whose radius is equal to 4 cm. Let P be a point whose distance from its
centre is 6 cm. Construct two tangents to it from P.
2