Page 4 - Lesson Note 1- ch 11 Division of line segment (Contruction)

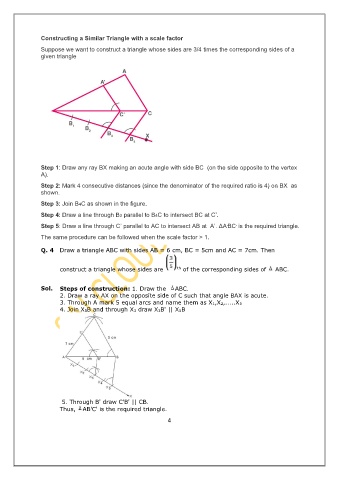
Constructing a Similar Triangle with a scale factor
Suppose we want to construct a triangle whose sides are 3/4 times the corresponding sides of a
given triangle

Step 1: Draw any ray BX making an acute angle with side BC (on the side opposite to the vertex
A).

Step 2: Mark 4 consecutive distances (since the denominator of the required ratio is 4) on BX as
shown.

Step 3: Join B4C as shown in the figure.
Step 4: Draw a line through B3 parallel to B4C to intersect BC at C’.

Step 5: Draw a line through C’ parallel to AC to intersect AB at A’. ΔA′BC′ is the required triangle.
The same procedure can be followed when the scale factor > 1.

Q. 4 Draw a triangle ABC with sides AB = 6 cm, BC = 5cm and AC = 7cm. Then

construct a triangle whose sides are th of the corresponding sides of ABC.

Sol. Steps of construction: 1. Draw the ABC.
2. Draw a ray AX on the opposite side of C such that angle BAX is acute.
3. Through A mark 5 equal arcs and name them as X1,X2,.....X5
4. Join X5B and through X3 draw X3B' || X5B

5. Through B' draw C'B' || CB.
Thus, AB'C' is the required triangle.
4