Page 2 - Lesson Notes- Ch-1 Decimal Expansions of Rational Numbers

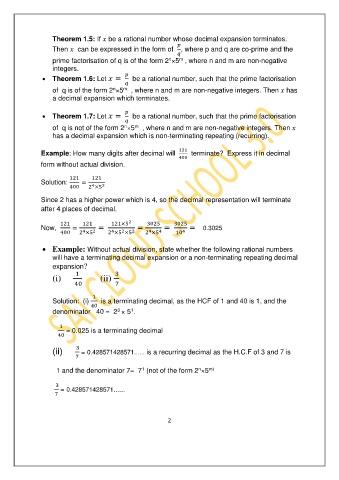
Theorem 1.5: If be a rational number whose decimal expansion terminates.

Then can be expressed in the form of , where p and q are co-prime and the

m
n
prime factorisation of q is of the form 2 ×5 , where n and m are non-negative
integers.

• Theorem 1.6: Let = be a rational number, such that the prime factorisation

of q is of the form 2 ×5 , where n and m are non-negative integers. Then has
m
n
a decimal expansion which terminates.

• Theorem 1.7: Let = be a rational number, such that the prime factorisation

m
n
of q is not of the form 2 ×5 , where n and m are non-negative integers. Then
has a decimal expansion which is non-terminating repeating (recurring).
121
Example: How many digits after decimal will terminate? Express it in decimal
400
form without actual division.

121 121
Solution: =
4
400 2 ×5 2
Since 2 has a higher power which is 4, so the decimal representation will terminate
after 4 places of decimal.

121 121 121×5 2 3025 3025
Now, = = = = = 0.3025
2
4
4
4
400 2 ×5 2 2 ×5 ×5 2 2 ×5 4 10 4
• Example: Without actual division, state whether the following rational numbers
will have a terminating decimal expansion or a non-terminating repeating decimal
expansion?
3
(i) 1 (ii)
40 7
1
Solution: (i) is a terminating decimal, as the HCF of 1 and 40 is 1, and the
40
1
3
denominator 40 = 2 × 5 .
1
= 0.025 is a terminating decimal
40

3
(ii) = 0.428571428571...... is a recurring decimal as the H.C.F of 3 and 7 is
7
1 and the denominator 7= 7 (not of the form 2 ×5
n
m)
1
3
= 0.428571428571......
7

2