Page 1 - Worksheet-1 Zeroes ,Remainder Theorem

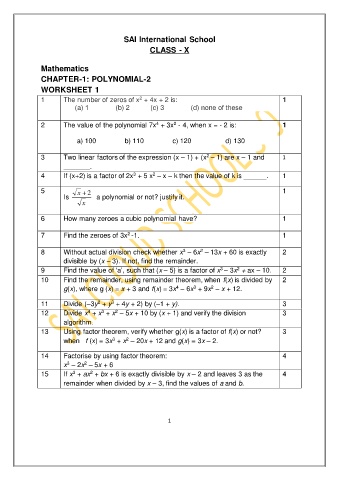
SAI International School
CLASS - X

Mathematics
CHAPTER-1: POLYNOMIAL-2

WORKSHEET 1
2
1 The number of zeros of x + 4x + 2 is: 1
(a) 1 (b) 2 (c) 3 (d) none of these

4
2
2 The value of the polynomial 7x + 3x - 4, when x = - 2 is: 1

a) 100 b) 110 c) 120 d) 130

2
3 Two linear factors of the expression (x – 1) + (x – 1) are x – 1 and 1
_______.
2
4 If (x+2) is a factor of 2x + 5 x – x – k then the value of k is ______. 1
3
5 x + 2 1
Is a polynomial or not? justify it.
x

6 How many zeroes a cubic polynomial have? 1

2
7 Find the zeroes of 3x -1. 1
3
2
8 Without actual division check whether x – 6x – 13x + 60 is exactly 2
divisible by (x – 3). If not, find the remainder.
9 Find the value of ‘a’, such that (x – 5) is a factor of x – 3x + ax – 10. 2
3
2
10 Find the remainder, using remainder theorem, when f(x) is divided by 2
4
2
3
g(x), where g (x) = x + 3 and f(x) = 3x – 6x + 9x – x + 12.
3
2
11 Divide (–3y + y + 4y + 2) by (–1 + y). 3
2
12 Divide x + x + x – 5x + 10 by (x + 1) and verify the division 3
3
4
algorithm.
13 Using factor theorem, verify whether g(x) is a factor of f(x) or not? 3
2
3
when f (x) = 3x + x – 20x + 12 and g(x) = 3x – 2.
14 Factorise by using factor theorem: 4
2
3
x – 2x – 5x + 6
3
15 If x + ax + bx + 6 is exactly divisible by x – 2 and leaves 3 as the 4
2
remainder when divided by x – 3, find the values of a and b.

1