Page 1 - LESSON NOTE

CHAPTER-12 THREE DIMENSIONAL GEOMETRY

LESSON NOTES

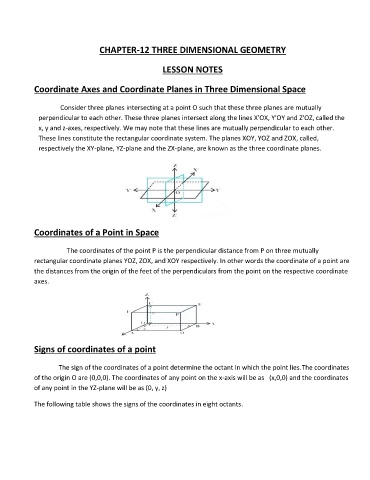
Coordinate Axes and Coordinate Planes in Three Dimensional Space

Consider three planes intersecting at a point O such that these three planes are mutually
perpendicular to each other. These three planes intersect along the lines X′OX, Y′OY and Z′OZ, called the
x, y and z-axes, respectively. We may note that these lines are mutually perpendicular to each other.
These lines constitute the rectangular coordinate system. The planes XOY, YOZ and ZOX, called,
respectively the XY-plane, YZ-plane and the ZX-plane, are known as the three coordinate planes.

Coordinates of a Point in Space

The coordinates of the point P is the perpendicular distance from P on three mutually
rectangular coordinate planes YOZ, ZOX, and XOY respectively. In other words the coordinate of a point are
the distances from the origin of the feet of the perpendiculars from the point on the respective coordinate
axes.

Signs of coordinates of a point

The sign of the coordinates of a point determine the octant in which the point lies.The coordinates
of the origin O are (0,0,0). The coordinates of any point on the x-axis will be as (x,0,0) and the coordinates
of any point in the YZ-plane will be as (0, y, z)

The following table shows the signs of the coordinates in eight octants.